1. KINEMATIC WAVE CELERITY

1.01
The kinematic wave celerity is a fundamental concept in engineering hydrology.

1.02
The kinematic wave celerity is the slope of the discharge-area rating:

1.03

1.04
Therefore:

1.05

1.06
A value of β = 5/3 is applicable to a hydraulically wide channel governed by Manning friction.

1.07
The kinematic wave celerity is also known as the Kleitz-Seddon, or Seddon, law.

1.08
In 1900, Seddon published a paper on unsteady flow movement in rivers, including flood waves.

1.09
Seddon's law is:

1.10

1.11
in which c = celerity of flood waves, Q = discharge, y = stage, and T = channel top width.

1.12
In open-channel hydraulics, the change in flow area is equal to the product of top width times the change in stage:

1.13

1.14
It follows that Seddon's law is a statement of the kinematic wave celerity.

1.15
The kinematic wave celerity is a function of the slope of the discharge-stage rating.

1.16
This slope is likely to vary with stage; therefore, the kinematic wave celerity is not constant, varying with flow level.

1.17
Therefore, in general, the kinematic wave equation is a nonlinear partial differential equation, requiring an iterative solution.

1.18
Theoretical values of β other than 5/3 can be obtained for other frictional formulations and cross-sectional shapes.

1.19
For turbulent Manning flow in typical channels, β has an upper value of 5/3.

1.20
For laminar flow in hydraulically wide channels, β = 3.

1.21
For mixed laminar-turbulent flow, β ranges from 5/3 to 3.

1.22
For hydraulically wide channels with Chezy friction, β = 3/2.

2. EXAMPLE

2.01
The kinematic wave celerity varies with friction and cross-sectional shape.

2.02
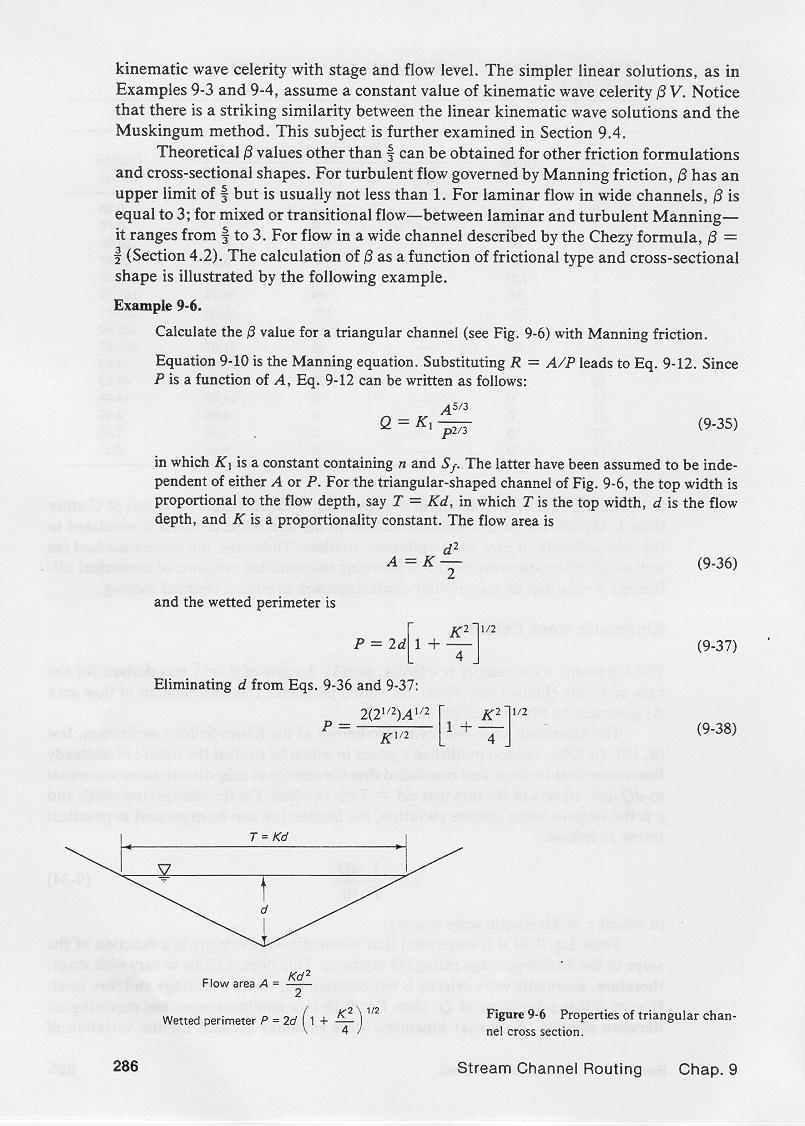
To illustrate, we calculate here the value of β for a triangular channel with Manning friction.

2.03
The Manning equation is:

2.04

2.05
Substituting into this equation the expression for hydraulic radius R = A/P:

2.06

2.07
Simplifying:

2.08

2.09
in which K₁ is a constant containing n and S_f.

2.10
We assume n and S_f to be independent of either A or P.

2.11
For a triangular channel, the top width is proportional to the flow depth:

2.12

2.13
in which T = top width, d = flow depth, and K = a proportionality constant.

2.14
The flow area can be expressed in terms of flow depth as follows:

2.15

2.16
Likewise, the wetted perimeter in terms of flow depth is:

2.17

2.18
Eliminating d from these two equations, leads to:

2.19

2.20
from which we obtain:

2.21

2.22
in which K₂ is a constant containing K.

2.23
Substituting this equation into the discharge equation leads to:

2.24

2.25
in which K₃ = a constant containing K₁ and K₂.

2.26
Comparing this equation with the rating equation, we conclude that the value of β for a triangular channel with Manning friction is 4/3.

3. LATERAL INFLOW

3.01
Practical applications of stream channel routing often require the specification of lateral inflows.

3.02
These inflows could be either concentrated, as in the case of tributary flow at a point along the channel reach, or distributed along the channel, as with groundwater exfiltration or infiltration.

3.03
A mass balance in the control volume leads to:

3.04

3.05
Note that this equation has the additional source term on the right-hand side, that is, the lateral inflow per unit channel length q_L.

3.06
Thus, the kinematic wave equation with lateral inflow is:

3.07

3.08
For positive lateral inflow, there is tributary inflow, or baseflow, that is, channel exfiltration.

3.09
For negative lateral inflow, there are transmission losses, that is, channel infiltration.

4. APPLICABILITY

4.01
The kinematic wave celerity is a fundamental streamflow property.

4.02
Flood waves which approximate kinematic waves travel with the kinematic wave celerity and are subject to very little or no attenuation.

4.03
In practice, flood waves are kinematic if they are of long duration or travel on a channel of steep slope.

4.04
Criteria for the applicability of kinematic haves have been developed.

4.05
The stream channel criterion states that in order for a wave to be kinematic, if should satisfy the following inequality:

4.06

4.07
in which t_r is the time-of-rise of the inflow hydrograph, S_o is the bottom slope, V_o is the average velocity, d_o is the average flow depth, and N = a dimensionless number.

4.08
For 95% accuracy on one period of translation, a value of N = 85 in recommended.

4.09
In practice, a flood wave will be kinematic if either the time-or-rise or the bottom slope are large.

4.10
Thus, the kinematic wave model applies to: (1) slow-rising flood waves in large basins of mild relief, for which the hydrograph time-of-rise is usually long, and (2) overland flow, for which the bottom slope is typically steep.

4.11

Narrator: Victor M. Ponce

Music: Fernando Oñate

Editor: Flor Pérez

Copyright © 2011

Visualab Productions

All rights reserved