1. DIFFUSION WAVE EQUATION

1.01
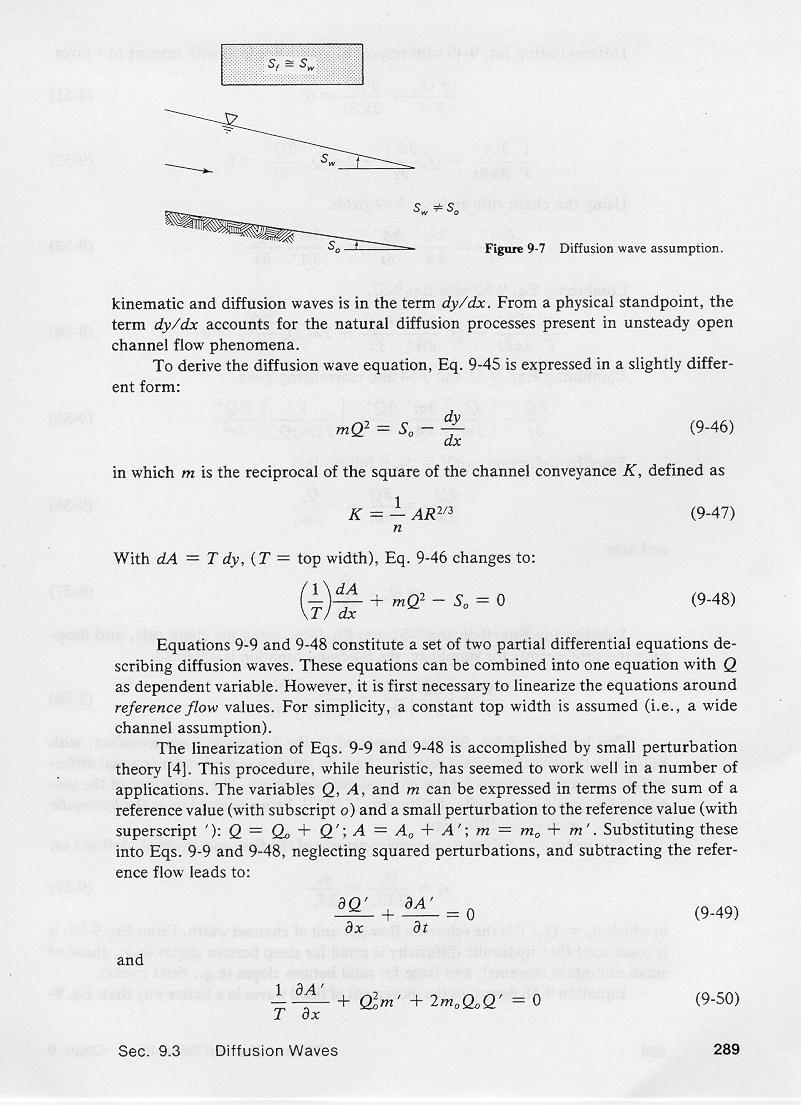
Kinematic wave theory can be enhanced by allowing a small amount of physical diffusion in its formulation.

1.02
In this way, an improved type of kinematic wave can be formulated, that is, a kinematic wave with diffusion, for short, a diffusion wave.

1.03
A definite advantage of the diffusion wave is that it includes the diffusion effect which is present in most natural unsteady open-channel flows.

1.04
The kinematic wave equation is derived using a statement of steady uniform flow in lieu of momentum conservation.

1.05
On the other hand, the diffusion wave equation is derived using a statement of steady nonuniform flow.

1.06
In other words, in the diffusion wave, the friction slope is equal to the water surface slope, rather than the bottom slope.

1.07
This leads to:

1.08

1.09
in which S_o is the bottom slope, and dy/dx is the flow depth gradient.

1.10
The difference between kinematic and diffusion waves is in the flow depth gradient.

1.11
From a physical standpoint, the flow depth gradient accounts for the natural diffusion process present in unsteady open-channel flow.

1.12
To derive the diffusion wave equation, the previous equation is expressed as follows:

1.13

1.14
in which m is the reciprocal of the square root of the conveyance K, defined as follows:

1.15

1.16
With:

1.17

1.18
This equation converts to:

1.19

1.20
The equation of continuity is:

1.21

1.22
These two equations constitute a set of two partial differential equations describing diffusion waves.

1.23
For certain applications, these equations can be combined into one equation with discharge Q as the dependent variable.

1.24
After appropriate linearization, the diffusion wave equation is obtained:

1.25

1.26
The left-hand side of this equation is recognized as the kinematic wave equation.

1.27
The right-hand side is a second-order term that accounts for the physical diffusion effect.

1.28
The coefficient of the diffusion term has the units L²/T.

1.29
This coefficient is referred to as Hayami's hydraulic diffusivity.

1.30
The hydraulic diffusivity is a characteristic of flow and channel, defined as follows:

1.31

1.32
in which:

1.33

1.34
is the reference flow per unit of channel width.

1.35
The hydraulic diffusivity is directly proportional to the unit-width discharge and inversely proportional to the channel slope.

1.36
Thus, diffusion is small for steep slopes and large for mild slopes.

1.37
Steep slopes are those of mountain streams, while mild slopes are typically those of large basins.

1.38
The diffusion wave equation describes the movement of flood waves in a better way than the kinematic wave.

1.39
It falls short of describing the full momentum effects, but it does physically account for wave attenuation.

1.40
The diffusion wave equation is a second-order differential equation of the parabolic type.

1.41
It can be solved analytically, leading to Hayami's diffusion analogy of flood waves.

1.42
It can also be solved numerically, using a scheme suited to parabolic equations such as the Crank-Nicolson scheme.

1.43
An alternate approach is to match the numerical diffusivity of the physical problem with the numerical diffusivity inherent in the Muskingum method.

1.44
This approach is the basis of the Muskingum-Cunge method.

2. APPLICABILITY

2.01
Most flood waves have a small amount of physical diffusion.

2.02
Therefore, they are better approximated by the diffusion wave rather than by the kinematic wave.

2.03
For this reason, diffusion waves apply to a much wider range of practical problems than kinematic waves.

2.04
When the diffusion wave fails, only the dynamic wave can properly describe the translation and diffusion of a flood wave.

2.05
However, the dynamic wave is very strongly dissipative, especially for flows well in the subcritical regime.

2.06
In practice, most flows are only mildly diffusive; therefore, they are subject to modeling with the diffusion wave.

2.07
A diffusion wave satisfies the following dimensionless inequality:

2.08

2.09
in which t_r is the time-of-rise of the inflow hydrograph, S_o is the bottom slope, d_o is the average flow depth, and g is the gravitational acceleration.

2.10
The greater the left-hand side of this inequality, the more likely that the flood wave is a diffusion wave.

2.11
In practice, a value of M = 15 is recommended for general use.

4.12

Narrator: Victor M. Ponce

Music: Fernando Oñate

Editor: Flor Pérez

Copyright © 2011

Visualab Productions

All rights reserved