OPEN-CHANNEL HYDRAULICS: LECTURE 031 - ENERGY PRINCIPLE

1. ENERGY PRINCIPLE
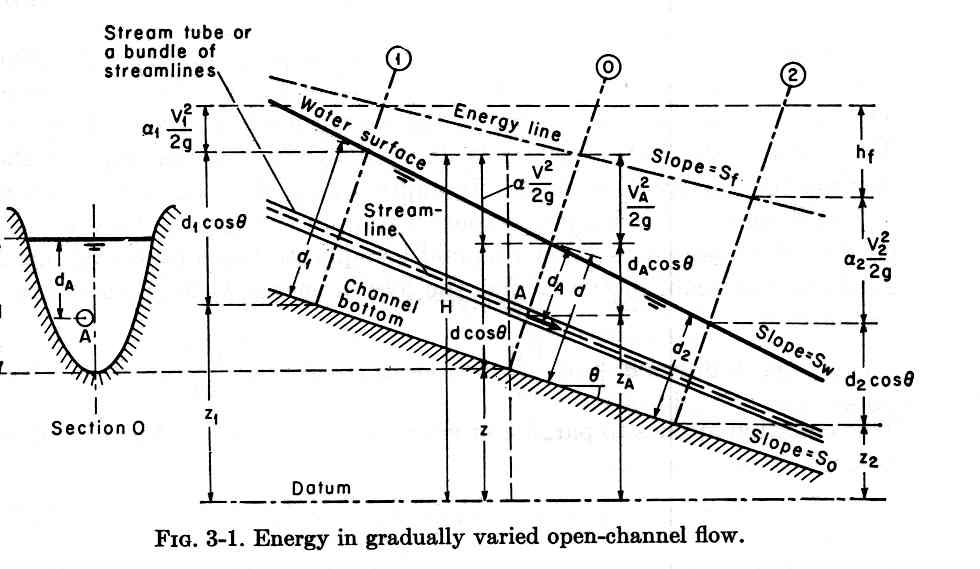
1.01
The total energy at point A on a streamline is:

                       v_A²
H = z_A + d_A cos θ + α

                       2g

Eq. 1

Fig. 01

Energy in gradually varied open-channel flow.

1.02
The total energy at a channel section is:

                      v²
H = z + d cos θ + α

                      2g

Eq. 2

1.03
The conservation of energy between sections 1 and 2 leads to:

               v₁²                 v₂²
z₁ + y₁ + α₁
= z₂ + y₂ + α₂
+ h_f
               2g                  2g

Eq. 3

1.04
in which h_f is the energy head loss.
1.05
The energy slope is:

       h_f
S_e =

       L

Eq. 4

1.06
in which L is the length of the channel reach.
1.07
The friction slope is:

       h_f'
S_f =

        L

Eq. 5

1.08
in which h_f' is the head loss computed based on the momentum principle.
1.09
Under steady flow, the friction slope is equal to the energy slope.

S_f = S_e

Eq. 6

1.10
Under uniform flow, the friction, energy, bottom, and water-surface slopes are all the same.

S_f = S_e = S_o = S_w

Eq. 7

2. SPECIFIC ENERGY
2.01
The concept of specific energy is useful in certain applications of open-channel flow.
2.02
The specific energy is the energy, per unit of weight, with reference to the channel bottom.

                 v²
E = d cosθ + α

                 2g

Eq. 8

2.03
For a hydraulically wide channel of mild slope, α = 1, and cos θ = 1. Then:

          v²
E = y +

          2g

Eq. 9

2.04
The statement of water continuity is:

Q = v A

Eq. 10

2.05
Therefore:

            Q²
E = y +

          2g A²

Eq. 11

2.06
The flow area is a function of flow depth:

A = f₁(y)

Eq. 12

2.07
Therefore:

             Q²
E = y +

          2g f₁(y)

Eq. 13

2.08
For a given discharge, the specific energy is a function of flow depth.

E = f₂(y)

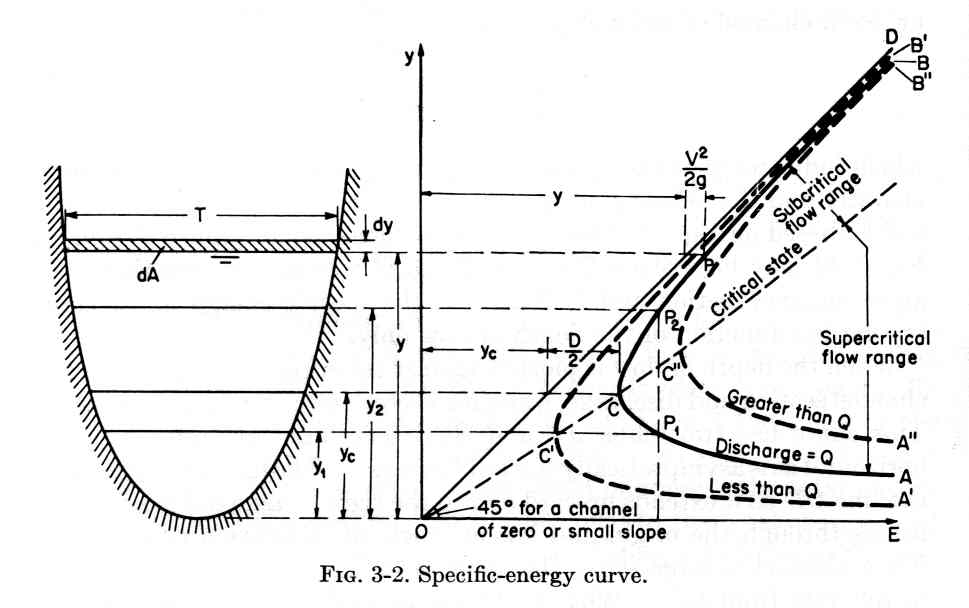
Eq. 14

2.09
This figure shows the specific energy curve, with discharge as a curve parameter.
Fig. 02

Specific energy curve.

2.10
The specific energy curve is a type of hyperbolic function.
2.11
Two values of flow depth correspond to a given value of specific energy.
2.12
One flow depth is small, and the other is large.

3. CRITICAL FLOW
3.01
The specific energy curve is a type of hyperbola and it has a minimum value of specific energy. This minimum value corresponds with critical flow.
3.02
The flow depth for critical flow is the critical flow depth.
3.03
A flow depth larger than critical corresponds to subcritical flow and subcritical depth.
3.04
A flow depth smaller than critical corresponds to supercritical flow and supercritical depth.
3.05
The minimum specific energy is obtained by taking the derivative of specific energy and equating to zero.

  dE          Q²   dA

= 1 -

= 0
  dy         gA³   dy

Eq. 15

3.06
The differential change in flow area is equal to the top width times the differential change in flow depth:

dA = T dy

Eq. 16

3.07
From which:

      dA
T =

      dy

Eq. 17

3.08
Therefore:

  Q² T

= 1
  g A³

Eq. 18

     V²

= 1
  g (A/T)

Eq. 19

  V²

= 1
  gD

Eq. 20

F² = 1

Eq. 21

3.09
That is, the condition of minimum specific energy is the square of the Froude number equal to 1.
3.10
Or, the Froude number equal to 1.

F = 1

Eq. 22

3.11
Also, at critical state, the velocity head is equal to half of the hydraulic depth.

  v_c²     D_c

=

  2g      2

Eq. 23

3.12
For a hydraulically wide channel:

  v_c²     y_c

=

  2g      2

Eq. 24

3.13
The statement of water continuity for critical flow in a hydraulically wide channel is:

q = v_c y_c

Eq. 25

3.14
Substituting lower case q into the critical flow equation:

  q²     y_c³

=

  2g     2

Eq. 26

3.15
And solving for critical depth:

        q²
y_c = [
]^1/3
        g

Eq. 27

3.16
Thus, in a hydraulically wide channel, the critical depth is solely a function of the unit-width discharge.

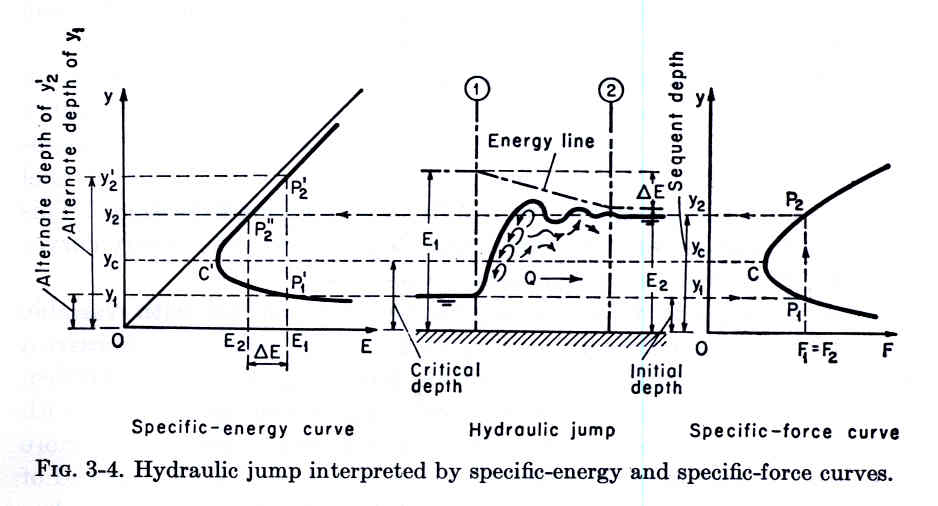
4. LOCAL PHENOMENA
4.01
Changes in the state of flow from subcritical to supercritical, and viceversa, can occur in open-channel flow.
4.02
Typically these changes occur within a relatively short distance, and the flow is rapidly varied. They are referred to as local phenomena.
4.03
The hydraulic jump and the hydraulic drop are local phenomena.
4.04
In a hydraulic jump, the flow changes from supercritical to subcritical flow.
4.05
A hydraulic jump occcurs when downstream flow conditions force the flow to change from supercritical upstream to subcritical downstream.
4.06
Flow inmediately downstream of an overflow spillway or sluice gate is a typical case of the ocurrence of a hydraulic jump.
Fig. 03

Hydraulic jump in Tinajones outlet canal, Lambayeque, Peru.

4.07
The jump occurs with a loss of energy.
4.08
The specific energy upstream of the jump is always greater than the specific energy downstream.
4.09
The difference between specific energies is the energy loss from the jump.
Fig. 06

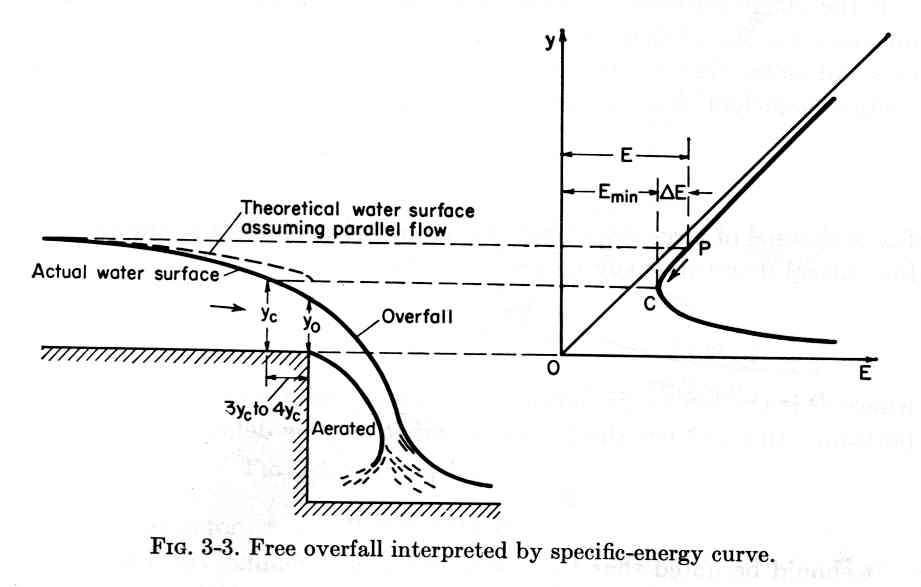
Hydraulic jump and specific energy.

4.10
In a hydraulic drop, the flow changes from subcritical to supercritical flow.
Fig. 04
Hydraulic drop in Tinajones feeder canal, Lambayeque, Peru.

4.11
The free overfall is a special case of hydraulic drop.
Fig. 05

Free overfall interpreted by specific energy curve.

4.12
However, the flow at the brink is actually curvilinear.
4.13
Therefore, the brink depth is not the critical depth as computed by the assumption of parallel flow.
4.14
The relation between critical depth and brink depth is:

y_c = 1.4 y_o

Eq. 28

4.15
in which y_o is the depth at the brink.
4.16
The location of critical depth is about 3 to 4 times the critical depth, measured from the brink in the upstream direction.

Fig. 01

Energy in gradually varied open-channel flow.

Fig. 02

Specific energy curve.

Fig. 03

Hydraulic jump in Tinajones outlet canal, Lambayeque, Peru.

Fig. 04

Hydraulic drop in Tinajones feeder canal, Lambayeque, Peru.

Fig. 05

Free overfall interpreted by specific energy curve.

Fig. 06

Hydraulic jump and specific energy.

Narrator: Victor M. Ponce
Music: Fernando Oñate
Editor: Flor Pérez

Copyright © 2011
Visualab Productions
All rights reserved