QUESTIONS

  1. What conservation laws are used in the description of steady gradually varied flow?

    Steady mass and energy are used in the description of steady gradually varied flow.

  2. What conservation laws are used in the description of unsteady gradually varied flow?

    Unsteady mass and momentum are used in the description of unsteady gradually varied flow.

  3. What forces are acting in a control volume in unsteady gradually varied flow?

    Four forces: (1) gravitational force, (2) frictional force, (3) the force due to the pressure gradient, and (4) inertia force.

  4. What waves types are common in unsteady open-channel flow?

    Five wave types: (1) kinematic wave, (2) kinematic-with-diffusion wave, (3) dynamic wave, (4) steady-dynamic wave, and (5) mixed kinematic-dynamic wave.

  5. To what problems do kinematic waves apply?

    Kinematic waves apply to overland flow and to steep channels, generally greater than 1% slope.

  6. To what problems do diffusion waves apply?

    Diffusion waves apply to flood routing in streams and rivers, with intermediate bottom slopes (0.01 > So > 0.0001).

  7. How is Lo defined?

    Lo is defined as ho/So, i.e., it is the length of channel that it takes the uniform flow to drop a head equal to its depth.

  8. What is the dimensionless wavenumber?

    The dimensionless wavenumber is the wavenumber 2π/L made dimensionless by multiplying it by Lo.

  9. What is the dimensionless relative celerity of kinematic waves under Chezy friction in hydraulically wide channels?

    The dimensionless relative celerity of kinematic waves under Chezy friction in hydraulically wide channels is 0.5.

  10. What is the dimensionless relative celerity of dynamic waves under Chezy friction in hydraulically wide channels?

    The dimensionless relative celerity of dynamic waves under Chezy friction in hydraulically wide channels is the reciprocal of the Froude number.

  11. What are three ways of expressing the convective celerity of kinematic waves?

    There are three ways of expressing the convective celerity of kinematic waves: (1) βV, (2) dQ/dA, and (3) (1/T ) dQ/dy.

  12. What is the neutral-stability Froude number for triangular channels with Chezy friction?

    The neutral-stability Froude number for triangular channels with Chezy friction is Fns = 4.

  13. What is the neutral-stability Froude number for hydraulically wide channels with Manning friction?

    The neutral-stability Froude number for triangular channels with Chezy friction is Fns = 1.5.

  14. What is the basis of the Muskingum-Cunge method?

    The matching of the physical diffusivity of the diffusion wave with the numerical diffusivity of the Muskingum-type numerical analog of the kinematic wave equation.

  15. What is Hayami's contribution to flood routing?

    Hayami developed the diffusion analogy of flood waves, by which flood waves are calculated using a convection-diffusion equation.

  16. For what value of Vedernikov number are kinematic and dynamic hydraulic diffusivities the same?

    For V = 0, i.e., for very low Froude number flows.

  17. For what value of Vedernikov number does the dynamic hydraulic diffusivity vanish?

    For V = 1.

  18. What part of the flood routing does the Muskingum parameter X account for?

    The parameter X accounts for the storage portion of the routing. For a given flood event, there is a value of X for which the storage in the calculated outflow hydrograph matches that of the measured outflow hydrograph.

  19. What is numerical diffusion?

    Numerical diffusion is a diffusion in the results, which is attributable to the first-order error due to the discrete (finite) grid.

  20. What is numerical dispersion?

    Numerical dispersion is a dispersion in the results, which is attributable to the second-order error due to the discrete (finite) grid.

  21. What is the advantage of the Muskingum-Cunge method?

    A unique feature of the Muskingum-Cunge method, which sets it apart from other numerical kinematic wave models, is the grid independence of the calculated outflow hydrograph. If numerical dispersion is minimized, the calculated outflow at the downstream end of a channel reach will be essentially the same, regardless of how many subreaches are used in the computation.

  22. When will the Muskigum-Cunge method fail to give good results?

    For the Muskingum-Cunge method to improve on the Muskingum method, it is necessary that the routing parameters evaluated at channel cross sections be representative of the channel reach under consideration. If this is not the case, the results may not be accurate.

  23. How is the downstream boundary specified in a mixed kinematic-dynamic model if increased accuracy is desired?

    By artificially extending the channel several subreaches downstream, and specifying a kinematic rating at the newly defined downstream boundary, while giving the loop a chance to develop at the upstream cross section of interest.

PROBLEMS

  1. You are observing the rise of a river during flood. The width of the river at the observation point, and for some distance upstream is 65 m, and according to the gage reading, the discharge is Q = 70 m3/s. Estimate the discharge at a point located 15.875 km upstream, if the water surface is rising at the rate of 9 mm/hr at your location and at 12 mm/hr at the upstream cross section.

    (∂Q / ∂x) + (∂A / ∂t) = 0

    (∂Q / ∂x) + B (∂y / ∂t) = 0

    r = ∂y/∂t

    (∂Q / ∂x) + B r = 0

    Qd - Qu = - B (Δx) r

    Qu = Qd + B (Δx) r

    Average r = 10.5 mm/hr

    Qu = 70 m3/s + 65 m (15,875 m) (10.5 mm/hr / 3600 s/hr) (1 m / 1000 mm)

    Qu = 73 m3/s  ANSWER.

  2. A hydraulically wide channel is operating at Froude number F = 0.22. The unit-width discharge is q = 2.8 m2/s. What are the two absolute Lagrange celerities?

    F = v / (gy) 1/2 = 0.22

    v = 0.22 (gy) 1/2

    F 2 = v 2 / (gy)

    F 2 = q 2 / (gy3)

    y = [ q 2 / (gF 2) ] 1/3

    y = [ 2.82 / (9.806 × 0.22 2) ] 1/3 = 2.547 m.

    cr = (gy)1/2 = (9.806 × 2.547) 1/2 = 4.998 m/s

    v = 0.22 (gy)1/2

    v = 0.22 × 4.998 = 1.099 m/s

    cd = v + cr = 1.099 + 4.998 = 6.097 m/s  ANSWER.

    cu = v - cr = 1.099 - 4.998 = - 3.899 m/s  ANSWER.

  3. A flood wave is traveling inbank through a straight river reach of top width T = 320 m wide and length L = 5625 m. For every 1 cm of flood rise, the discharge goes up 10 m3/s. How long will it take for a given discharge to travel the length of the reach?

    Use the Seddon formula for flood wave speed (celerity):

    c = (1/T) dQ / dy

    c = (1/T) ΔQ / Δy

    Assume Tt = travel time through the reach.

    c = L / Tt

    L / Tt = (1/T ) ΔQ / Δy

    Tt = L T (Δy / ΔQ )

    Tt = (5625 m) (320 m) (0.01 m) / (10 m3/s)

    Tt = 1800 seconds  ANSWER.

    Tt = 30 minutes  ANSWER.

  4. Calculate the exponent β of the discharge-area rating for a triangular channel with Chezy friction.

    Q = C A R1/2 S1/2

    Q = C A (A1/2 / P1/2) S1/2

    Q = C (A3/2 / P1/2) S1/2

    Q = K1 A3/2 / P1/2

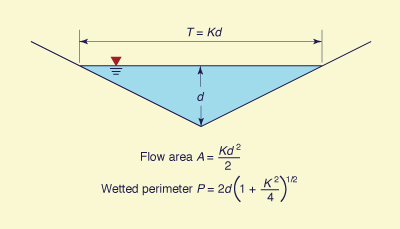
    T = Kd

    A = Kd 2 / 2

    P = 2d [ 1 + (K 2/4) ]1/2

    d = (2A / K )1/2

    P = 2 (2A / K )1/2 [ 1 + (K2/4) ]1/2

    P = 2 (2 / K )1/2 [ 1 + (K 2/4) ]1/2 A1/2

    P1/2 = K2 A1/4

    Q = K1 A3/2 / (K2 A1/4)

    Q = K3 A5/4

    β = 5/4  ANSWER.

    Definition sketch for a triangular channel

    Fig. 10-23  Definition sketch for a triangular channel.

  5. Calculate the exponent β of the discharge-area rating for a triangular channel with Manning friction.

    Q = (1/n) A R2/3 S1/2

    Q = (1/n) A (A2/3 / P 2/3) S1/2

    Q = C (A5/3 / P 2/3) S1/2

    Q = K1 A5/3 / P 2/3

    T = Kd

    A = Kd 2 / 2

    P = 2d [ 1 + (K 2/4) ]1/2

    d = (2A / K )1/2

    P = 2 (2A / K )1/2 [ 1 + (K2/4) ]1/2

    P = 2 (2 / K )1/2 [ 1 + (K 2/4) ]1/2 A1/2

    P 2/3 = K2 A1/3

    Q = K1 A5/3 / (K2 A1/3)

    Q = K3 A4/3

    β = 4/3  ANSWER.

  6. A flood hydrograph has the following data: time-of-rise tr = 2 hr, reference velocity Vo = 2 ft/s, reference flow depth do = 6 ft, bottom slope So = 0.004. Determine is this wave is a kinematic wave.

    tr So Vo / do = (2 × 3600) (0.004) (2) / (6) = 9.6 < 85

    This wave is NOT a kinematic wave.  ANSWER.

  7. A flood hydrograph has the following data: time-of-rise tr = 2 hr, reference velocity Vo = 2 ft/s, reference flow depth do = 6 ft, bottom slope So = 0.004. Determine is this wave is a diffusion wave.

    tr So (g / do)1/2 = (2 × 3600) (0.004) (32.17 / 6)1/2 = 66.7 > 15

    This wave is a diffusion wave.  ANSWER.

  8. A flood hydrograph has the following data: time-of-rise tr = 1 hr, reference velocity Vo = 2 m/s, reference flow depth do = 2 m, bottom slope So = 0.0004. Determine is this wave is a diffusion wave.

    tr So (g / do)1/2 = (1 × 3600) (0.0004) (9.806 / 2)1/2 = 3.18 < 15

    This wave is NOT a diffusion wave.  ANSWER.

  9. Using ONLINE ROUTING 04, route a flood wave using the Muskingum method. The inflow hydrograph ordinates are [25 ordinates, starting at time = 0, to time = 24 hr]:

    100, 130, 150, 180, 220, 250, 300, 360, 450, 550, 700, 550, 490, 370, 330, 310, 280, 230, 170, 150, 130, 120, 110, 105, 100.

    Assume Δt = 1 hr, K = 1 hr, X = 0.3. Report the peak outflow and time of occurrence.

    From the output table, the peak outflow is 651.46 m3/s, and it occurs at time t = 11 hr.  ANSWER.

  10. Using ONLINE ROUTING 05, route the same flood wave as the previous problem using the Muskingum-Cunge method. Use the following input data: Qp = 700 m3/s, Ap = 400 m2, Tp = 88 m, Δt = 1 hr, Δx = 9.6 km, β = 1.65, So = 0.0007. Report the peak outflow and time of occurrence.

    From the output table, the peak outflow is 652.994 m3/s, and it occurs at time t = 11 hr.  ANSWER.

http://openchannelhydraulics.sdsu.edu
140821 10:05

Documents in Portable Document Format (PDF) require Adobe Acrobat Reader 5.0 or higher to view; download Adobe Acrobat Reader.