CIV E 445 - APPLIED HYDROLOGY
SPRING 2014
HOMEWORK No. 12 SOLUTION
The total rainfall depth is: P = 16 cm. With CN = 81, and R = 2.54 cm/in, the effective rainfall depth (i.e., runoff) (Eq. 5-9) is: Q = 10.56 cm.
Assuming φ between 0 and 1 cm/h: [(1 - φ) • 1 + (2 - φ) • 1 + (5 - φ) • 1 + (4 - φ) • 1 + (3 - φ) • 1 + (1 - φ) • 1] = 10.56.
From which: φ = 0.9067 cm/h. The total and effective rainfall pattern is:
Time (h) Total rainfall φ-index Effective rainfall 0-1 1 0.9067 0.0933 1-2 2 0.9067 1.0933 2-3 5 0.9067 4.0933 3-4 4 0.9067 3.0933 4-5 3 0.9067 2.0933 5-6 1 0.9067 0.0933 Total 16 - 10.5598
≅ 10.56The accumulated effective rainfall depth is: 10.5598 cm.
The total runoff volume is: 85 km2 • 10.5598 cm = 897.583 km2-cm = 8.97583 hm3.
The calculations are shown in the following table:
Time
(hr)Time-area histogram subareas
(km2)Partial flows (km2-cm/hr)
for indicated rainfall incrementsOutflow
(km2-cm/hr)Outflow
(m3/s)0.0933
cm/hr1.0933
cm/hr4.0933
cm/hr3.0933
cm/hr2.0933
cm/hr0.0933
cm/hr0 - 0 - - - - - 0 0 1 17 1.5861 0 - - - - 1.5861 4.40 2 21 1.9593 18.5861 0 - - - 20.5454 57.07 3 31 2.8923 22.9593 69.5861 0 - - 95.4377 265.10 4 16 1.4923 33.8923 85.9593 52.5861 0 - 173.9305 483.14 5 - 0 17.4923 126.8923 64.9593 35.5861 0 244.9305 680.36 6 - - 0 65.4928 95.8923 43.9593 1.5861 206.9305 574.81 7 - - - 0 49.4928 64.8923 1.9593 116.3444 323.18 8 - - - - 0 33.4928 2.8923 36.3851 101.07 9 - - - - - 0 1.4928 1.4928 4.15 10 - - - - - - 0 0 0 Sum 85 - - - - - - 897.583 -
The sum of outflow hydrograph ordinates is 897.583 km2-cm/h.
The integration of the outflow hydrograph results in: 897.583 km2-cm/h • 1 h = 897.583 km2-cm = 8.97583 hm3, which is the same as the total runoff volume. The same results obtained with ONLINE ROUTING 06 (shown below). ANSWER.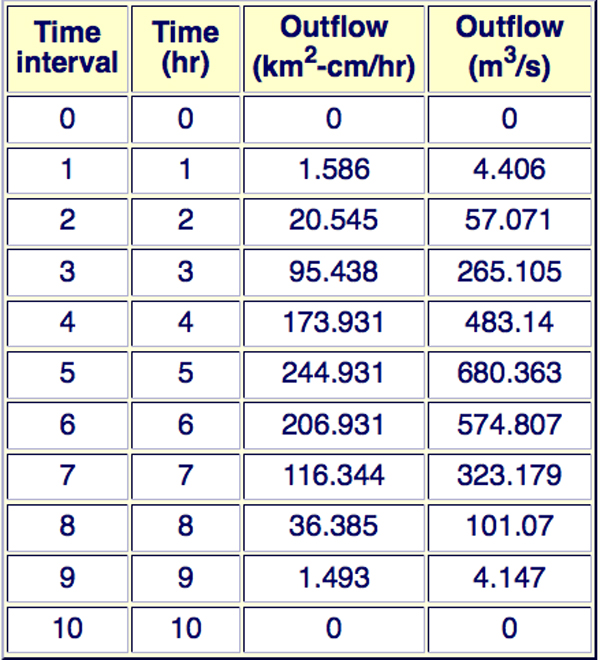
- Since Δt = 1 h, and K = 3 h: Δt /K = 1/3, and from Eq. 8-16 to 8-18, the linear reservoir-routing coefficients are the following:
C0 = 1/7; C1 = 1/7; C2 = 5/7.
The unit runoff volume is: (20 + 40 + 60 + 40 + 24 + 16) km2 • 1 cm = 200 km2-cm = 2 hm3.
Since the duration of the SI unit hydrograph (1 cm of runoff) is 2 h, the rainfall intensity is 0.50 cm/h.
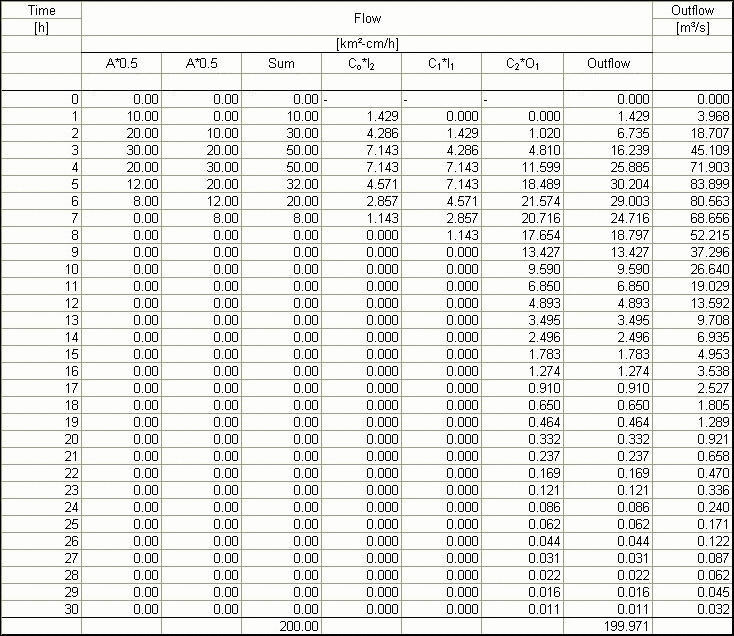
The peak outflow is 83.899 m3/s, and it occurs at time = 5 hr.
The same results obtained with ONLINE ROUTING 07. ANSWER.
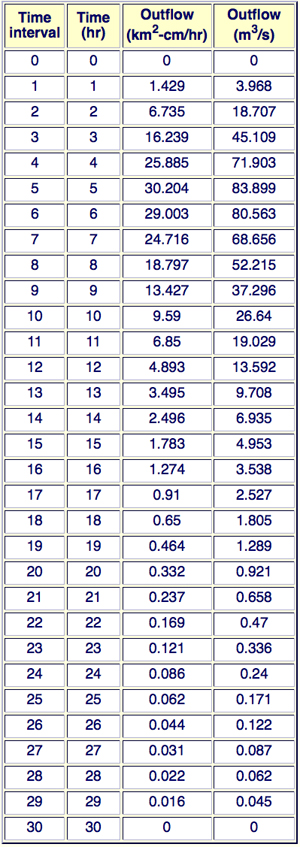
The results for Example 10-3 are given below, for K = 12 hr, and N = 3. The peak outflow is 308.611 km2-cm/hr, or 857.253 m3/s, and it occurs at time = 36 hr.
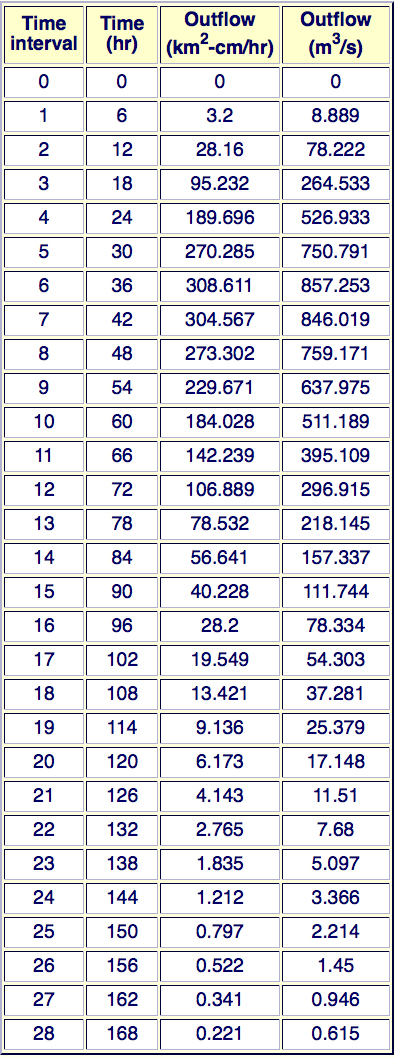
The results for K = 12 hr, and N = 4 are shown below. The peak outflow is 723.115 m3/s, and it occurs at time = 48 hr.
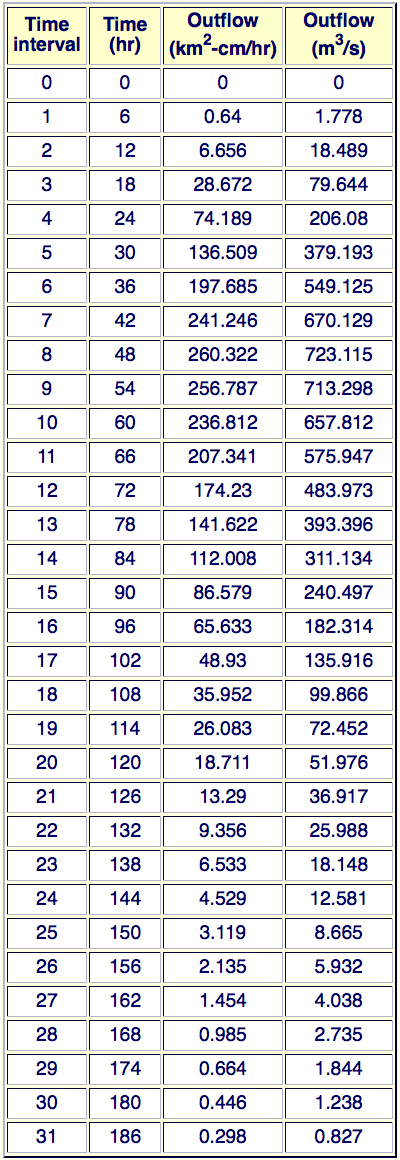
The results for K = 12 hr, and N = 5 are shown below. The peak outflow is 429.853 m3/s, and it occurs at time = 84 hr.
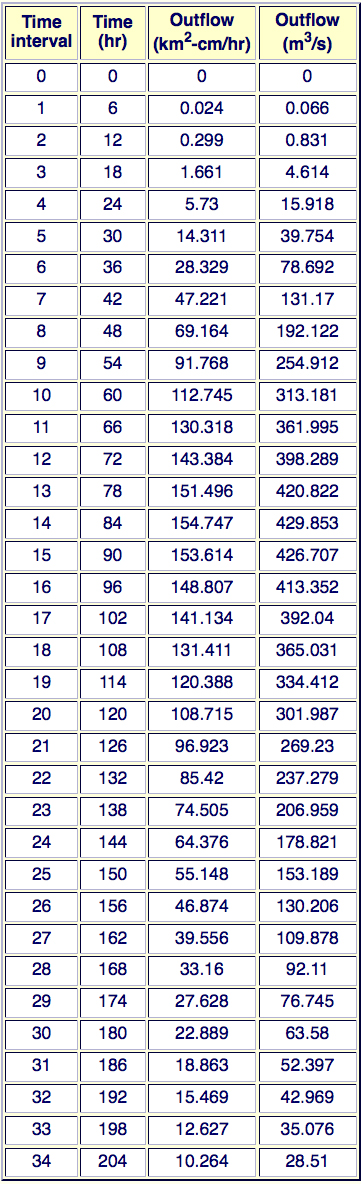
As K and/or N increase, the peak outflow is reduced and the time-to-peak increases, as expected.
The time of concentration is: tc = 6 hr.
The effective rainfall duration for this unit hydrograph is: tr = 1 hr.
The time base of the translated-only hydrograph (Eq. 10-4) is:
Tb = tc + tr = 7 hr.
The evaluation of K can be based on Eq. 10-4, using two consecutive ordinates after 7 hr.
Using Eq. 10-4 and the ordinates at 7 hr and 8 hr:
K = - [(47 + 28) / 2] / [(28 - 47) / 1] = 1.97 hr.
Since this a unit hydrograoph derived from data, it may be appropriate to average several values of K computed at the tail of the hydrograph. Using the ordinates at 8 and 9 hr: K = 2.04 hr. Using the ordinates at 9 and 10 hr: K = 1.92 hr. Using the ordinates at 10 and 11 hr: K = 2.00 hr.
The average of four values is: K = 1.98 hr, say K = 2 hr.